Last Update: May 3rd, 2015

• Brampton Booster
• May 03, 2015
• Comment

Recently Added

• The inherent power of Stride units
• How to stoop to a bad player’s level
• Current and future goals for this blog
• How to overkill
• Chaos in vanguard

Topics are presented in a very sporadic manner. I suggest using RSS feeds (or the mailing list I’m trying to set up) in order to keep up to date. In terms of how “updated” I am about the scene I would say a whole lot. Even though I lack articles on Legion or Stride or the next booster set I do a fair bit of research on everything. My blog tends to look more into the foundations rather then the next big thing so it often seems like I’m behind but rest assured. If you have anything you want to specifically point out then I’ll provide a preliminary answer as fast as possible. Articles take a lot of time to write, cross-reference, and articulate. That said, my list for future projects include:

• Early game optimization
• Attacking with a locked/locked-down field
• Determinate dominating decks

Stride Probabilities

• Brampton Booster
• May 03, 2015
• Comment

In a year from now, anyone picking up vanguard for the first time would believe that Stride was a mechanic from the very beginning. Unlike other card games which shove in a mechanic for the sake of novelty, Bushiroad shows that when it wants to create good design they succeed very well.

Vanguard has always pushed for quality over quantity, by allowing each resource to be used in multiple ways¹. The Deck can be drawn and stacked, and the Drop Zone can aid in Legion. The Damage Zone was the most ingenious by having triggers, counter blasts, unflipped, limit breaks, card swapping, and card inclusions maximizing its utility. So the G-Zone had to be more then just an extra deck. This was a achieved by having it work in a reverse Damage Zone; cards are flipped face up and face up cards contributed to G-breaking/counting. This is why stride fits so well into the current game and honestly, should have been in the game at the very start.

But how has Stride changed the game we play today? Do the triple checks and card cycling push the game too far? Or has Vanguard’s Day 0 future-proofing technology shown us what it truly means to have brilliant game design?

G-Assist really works

Surprising nobody, G-Assist has fixed many complaints about being grade locked. But by how much did it really help? By calculating the difference with and without G-assist, basic ride success have been fixed by at least 14%. Below is the output of the results:

G Assisted	Normal	Difference	Grade 1	Grade 2	Grade 3
0.96495	0.82942	0.13553	12	11	10
0.96343	0.82595	0.13748	11	12	10
0.96302	0.82416	0.13885	11	11	11
0.96262	0.82629	0.13633	12	12	9
0.96205	0.82579	0.13626	13	11	9
0.96198	0.82306	0.13892	12	10	11
0.96183	0.82434	0.13748	13	10	10
0.95943	0.81937	0.14006	11	13	9
0.95847	0.8157	0.14277	10	12	11
0.95836	0.81433	0.14404	11	10	12

And the ranking without G-Assist

G Assisted	Normal	Difference	Grade 1	Grade 2	Grade 3
0.82942	0.96495	-0.13553	12	11	10
0.82629	0.96262	-0.13633	12	12	9
0.82595	0.96343	-0.13748	11	12	10
0.82579	0.96205	-0.13626	13	11	9
0.82434	0.96183	-0.13748	13	10	10
0.82416	0.96302	-0.13885	11	11	11
0.82306	0.96198	-0.13892	12	10	11
0.81937	0.95943	-0.14006	11	13	9
0.8157	0.95847	-0.14277	10	12	11
0.81433	0.95836	-0.14404	11	10	12

An important fact is that the top grade ratios are different whether you compare by G-Assist or not. Nonetheless, The best grade ratio is the same for both tables. By ranking the G-Assist changes the ranking like this: 1, 3, 6, 2, 4, 7, 5, 8, 9, 10².

We can compare it to the best ride chain (Stern) to see improvements in that:

G Assisted	Normal	Difference	Grade 1	Grade 2	Grade 3
0.97029	0.8594	0.11089	13	10	10
0.97008	0.86256	0.10752	13	9	11
0.96962	0.85748	0.11215	12	10	11
0.9691	0.8612	0.1079	14	9	10
0.96822	0.85188	0.11633	12	11	10
0.96781	0.8572	0.11061	12	9	12
0.96664	0.85318	0.11346	14	10	9
0.96648	0.86118	0.1053	14	8	11
0.96621	0.84895	0.11725	13	11	9
0.96588	0.85912	0.10676	13	8	12

Difference is smaller as expected. Ranking for type one ride chain 13+ grade 3’s looks like this:

Normal	G Assisted	Difference	Grade 1	Grade 2	Grade 3
0.85147	0.96274	-0.11127	12	8	13
0.84937	0.95735	-0.10798	13	7	13
0.84551	0.96233	-0.11681	11	9	13
0.84037	0.95386	-0.11349	12	7	14
0.83842	0.95688	-0.11846	11	8	14
0.83151	0.95633	-0.12482	10	10	13
0.82755	0.95321	-0.12566	10	9	14
0.82672	0.948	-0.12128	11	7	15
0.81984	0.94772	-0.12788	10	8	15
0.80922	0.94436	-0.13514	9	11	13

One needs to remember the cost of G-Assist though. A minus 1 in the early game can be fatal against certain decks. While you can go with a ridiculous 4-1-28³ with a 75% success rate it will cost you severely since you will G-Assist more then half the time. Since I can not phantom any Morikawa-type deck that requires such a ridiculous ratio (and a severe lack of grade 1 boosters), it would be much safer to stick with the pre-stride era rankings.

Stride increased cycle speed

There is a nice rule of thumb when checking how often a card would show up; by having it divided by the deck size.

• Perfect guards happen every 12 cards (49/4 ~= 12)
• Having a main[4] and backup[4] set keeps a cycle at 6 cards (49/8 ~= 6)
• Triggers will appear every 3 cards (49/16 ~= 3)

And there are two cycles pre-stride; the Mulligan and turn 3 onwards. The base tempo is 3 cards, one for drawing and another two with twin drive. It is expected to go through 1 other card by damage, and usually one more by advantage decks (RP,OTT,NN) making tempo around 4.5. The starting, Mulligan, and first draw goes through 9 cards which is double that. In the first couple of turns or past turn 7, tempo is only 2-3. From this data you can tell perfect guards are picked up every three turns while triggers pop up every single turn. However, there is a smaller chance to have a trigger activate in the right timing (2/4.5) while important cards have better odds (4/4.5). As the cycle normally continues about 5 times you can see how nicely the numbers line up.

When Stride came in vanguard suddenly became much faster. Although the beginning is still 9 cards, an average turn of striding is now 6. You draw, then triple check, G-skills activate, and you very often take damage. Triggers now have a 12% increase to (3/6) and key cards show up (5/6). This means perfect guards now move from every three turns to every two turns, and you get two triggers every cycle. So if you considered why stride had obvious power creep that didn’t seem to translate completely, it’s due to additional speed created in picking up defensive cards. However, that isn’t enough…

Heals are useless, 16 CRIT all the way

Before you label me as a lunatic please consider the data I have gathered on this. When I say heals are useless, I’m referring to a specific situation where both players are using vanguard with triple drive from turn 3 onwards. I mean, there is no such thing as a triple checking vanguard right?

Sarcasm aside, nobody is going to Stride for 6 turns straight. Whether it be by choice or by restriction (Too much G-flipping or can’t discard a grade 3) players will attack with grade 3’s. Now, as most of the decent finishers are either re-standing vanguards or pg-nullifying units heal triggers are both extremely valuable and a major hindrance. While the meta leans toward 12 CRIT, if Striding becomes cheaper and Strides become stronger the game might inherently move towards 16 CRIT⁴.

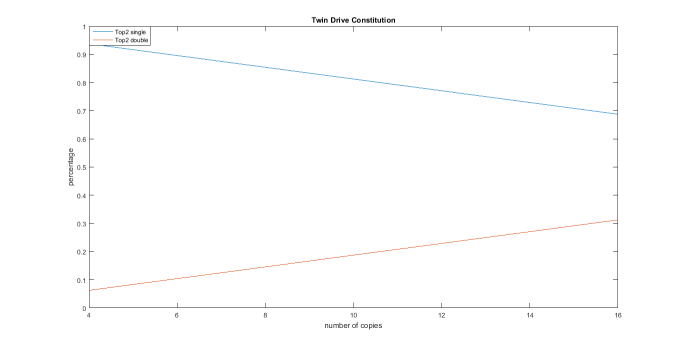
So, let’s assume this futuristic world then and map out probabilities for getting a crit in twin and triple drive:

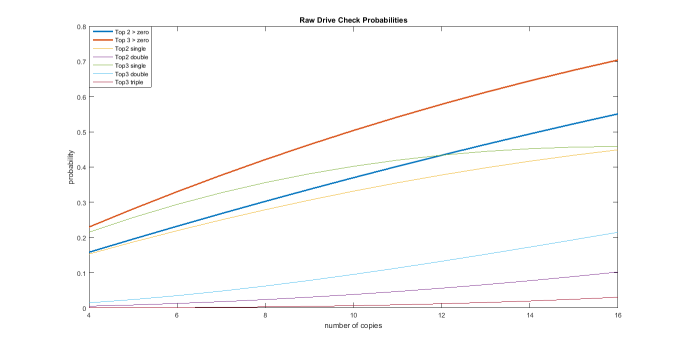
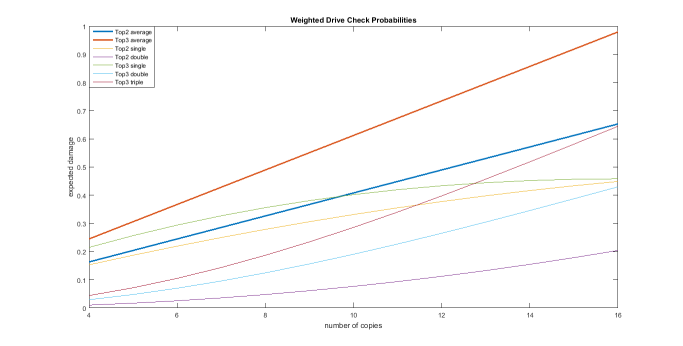
First of all none of these lines have a linear relationship but rather a hypergeometric one. This is a generic graph where we will base everything on. While it be for perfect guards or grade 3 the probabilities are the same meaning this chart can be used for further analysis. We are focusing on critical triggers for this article; The bold blue line is the chance of getting at least one crit in twin drive (referred to as /#/2 from now on) while the bold red one is for triple checks (/#/3). The lines may have the same shape but /#/3 has a slightly higher slope edging out at 0.7 while /#/2 is down at 0.55. This should be obvious as we are comparing twin drive to a triple drive. But these lines do not show to average amount of extra damage dealt per drive check. Getting two critical triggers mean 2 extra damage and three makes 3. So we need to weigh each probability to match the expected damage output which is reflected in Figure 2:

As calculated before, running 12 critical triggers increases the damage tempo from 1 to 1.5 per turn, having 8 is a 1 -> 1.33 and 16 is 1-> 1.66. One interesting fact is that /#/2’s drive tempo is linear meaning that triggers are in fact separated by ratios (1 + 0.5/12*8 = 1.3). This is also true for the triple check and as you can already see, it has an absolute dominance over everything. So if there is 16 critical triggers in your deck, the tempo of /16/3 is 2 damage per turn. Due to the linear relationship it means that 16 crit increases damage at twice the rate of 8 crits.

If you read through this argument a couple of times, you might realize that since tempo of /#/2 is also linear, the double tempo thing applies to /16/2 as well. But you fail to account two things:

1. 16 critical triggers do not increase true tempo to two damage per turn, and
2. Heal triggers exist to mitigate the damage dealt by extra critical values

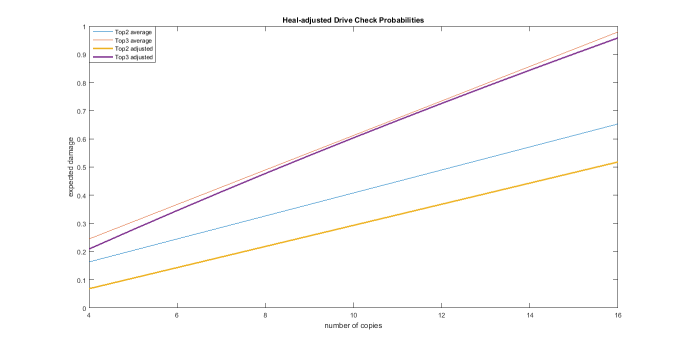
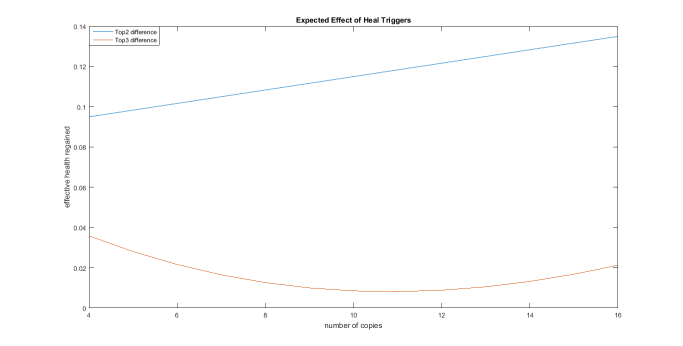
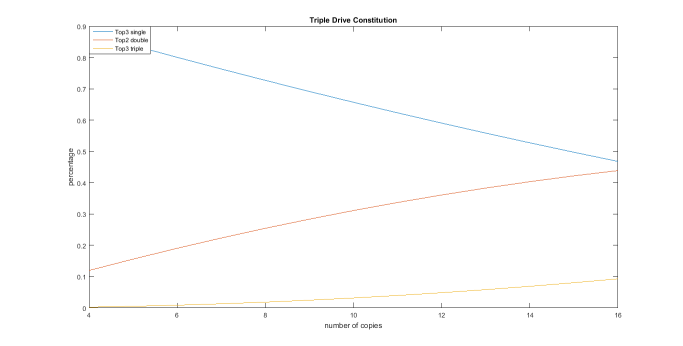
This is my strongest case for 16 crits. Look at the difference between /#/2 /#/2+adjusted. The difference is more then 10%. But between /#/3 and /#/3+ there is next to no difference. Figure 4 below calculates the actual difference and blows it up to make it easier to see. In //twin+, as you put more and more crits in your deck, the stronger the effect of heal triggers becomes. But this is not the case for /#/3+. In fact, the effect of heal triggers get weaker as you put more crits until you hit 12 copies. This concludes that /#/3 practically makes heal useless, which is what all my research has lead me.

It does not matter what number of criticals you use in your deck, Striding basically has a hidden CONT that screws over your opponent’s heals⁵. At the low end /4/3+ is 250% more effective then /4/2 while the other end /16/3 is 700% more effective then /16/2. Combined with strong linear slope /16/3 has you can force the game to move twice as fast and kill threaten death even at a normal 3 damage. But there is something more unsettling when drive composition comes into play.

We first show a deconstruction of the twin drive. As you can see it’s quite tame. You are more likely to get a single crit then two regardless of how many triggers you put in. Even at 16 copies, double crit only happens a third of the time. The actual probability to get it is actually one half of one third of two thirds, or 10%. When I mean a third, I mean the composition tells me that if I do get a crit on the first check I have a one in three chance for the other trigger to be a crit.

Nothing about the figure is particularly interesting, except at the very end. By extrapolating the number of triggers by one the lines actually cross and hence a higher chance of getting two criticals as opposed to just one. So let’s take in account 16 critical triggers again: In twin drive, a crit on the first check signifies a 33% for a second one. But in triple drive getting a crit on the first check implies a 55% chance to pick up another⁶! (Don’t forget the chance of a triple crit).

In terms of raw probabilities you are still going to miss getting a trigger 30% of the time. And if they guard for two to pass, it will still benefit them 60% of the time (Though for twin drive it works 90%). But be aware of your situation; if you get a crit and the guard wasn’t perfect you have a great chance to take them out right there.

Sticking with heals

To reiterate; If the meta changes over to triple checking vanguards 16 crit will be more effective then any other ratio. /16/3+ will force a damage tempo of 2, negate the power of heals, and has a high chance to break two-to-pass defending. If the opponent can not defend they get an average of two damage dealt: obviously this is accounting the weight of double and triple crits. They only get perfect guards one in three turns [HGCC(49,4,4,0,”>”)], and in this case the best decks will be pumping power so a rearguard can also swing for 5+ stages and 2+ crit (ideally). Speed is your friend: you want to keep both parties invested in Stride so you can reap all the benefits.

However twin drive is still a central part of vanguard and it isn’t going to leave peacefully. Breakrides and limit break decks in general now have a response to their stagnated mid-game power, while Legion finds another way to drop cards at a faster rate and protect it while the drop zone builds. Restanding vanguards that plus generously are still limited to twin drive, and of course the G-zone does have a set limit of 8. Lastly, stride requires a blood sacrifice and not every deck can provide them with a limitless of grade 3’s. When the fuel supply runs out, so is the advantage of /16/3.

Figure 4 (The one on expected effect of heals) wrap up the benefits of staying loyal to 12 Crit/4 Heal. If the opponent opts for /#/2+ you will have to tank the 0.33-0.5 damage tempo instead of the greatly reduced 0.22-0.4 pacing that you have been used to beforehand. And when combining both twin and triple drive, 12 crit fit right into the minimum of that curve while having a medium effect on heals. In symbolic terms, /#/2 > /#/3 for you <=> the opponent is /#/2.

Protection

I’ll close out with how effective future-proofing has done for vanguard. Stride is definitely a game-changing mechanic that causes us to re-evaluate a lot of different decks and situations that was often bad or marginal. It benefits every deck and improves consistency. Disregarding the ability to solve grade locking, the consistency has the added benefit of increasing tempo both offensively and defensively. While offense gets the better deal, there is still enough room to wriggle out of tight spots. Unfortunately the game is very close to tipping over to one side, so Buishiroad will need to find a way to improve a defenders turn before power levels become too high to defend properly. With my midgame standard form being less accurate I will lean towards early game optimization. Keep a good eye out so you can see if the metagame shifts to a /16/3 favoured build before the next game-changing mechanic arrives.

Sub-optimal strategies and Donkeyspace

• Brampton Booster
• Feb 21, 2015
• Comment

Optimal.
Optimal.
Optimal.

Do you think being optimal means that strategy is the best way to play? Does it trounce suboptimal plays each and every time? And if you said no, in what way would a sub-optimal play be preferred?

What Is Optimal

I think the largest misconception about optimization it that is it is “the best way to play”. But that’s not exactly what optimization means. In a theoretical sense, it means you play with the least exploitably. It means that regardless of what the opponent does, you won’t be at any larger disadvantage then if you used a suboptimal tactic. The problem with people solely grinding optimal strategies is that they leave out much of the mortal factors. Much of the time¹ we are facing opponents with suboptimal deck builds and suboptimal strategies and when confronted with that, sometimes it is necessary to also play suboptimally in order to exploit that weakness.

It is important to understand that optimal plays are based only on the situation, ignoring the human bias. A scenario:

• you are certain to lose next turn
• your opponent has a infinite wall of cards
• they decide to 2-pass your vanguard
• and you pulled a trigger on the first check

The optimal solution is to put everything on the vanguard. The sub-optimal solution is to pass it to a rearguard. You are playing in a way that provides you the lowest chance of losing or “playing least exploitably”. Other situations call for different optimal and suboptimal plays taking into account all the mechanics and knowledge about the specific situation.

Now let’s turn this around for a moment. If you had an infinite wall of cards, why did you decide to 2-pass? Clearly that is a suboptimal strategy that introduces a way to be exploited. But what if your hand was actually an infinite wall of grade 3’s, barring enough to defend the remaining two unboosted rearguard lanes? It would then be your optimal play to land a two-to-pass. At the same time, would the optimal solution for the opponent be to pass it to the rearguard? No! Remember that each individual has a different set of information and that being optimal only accounts for the information they acquired. Should you have learned their hand was full of grade 3’s, it would then be reasonable to say that would be optimal. Optimal plays are very local and are heavily dependant on individual scenarios.

Looks good on paper

Let’s imagine Rock Paper Scissors. Now imagine the opponent, say his name is Frank, randomly throws each option at the same probability with no bias (1/3,1/3,1/3). How would you play in order to get the highest chance of winning?

Let’s try throwing out 100% paper. In this case, you have 1/3 chance of winning, 1/3 chance of losing, and 1/3 of a tie.
Can we get better? Let’s try to use paper 50% of the time, and switch to scissors for the other half. Again, you are confronted with a 33,33,33 spilt. In fact, regardless of what you try to do it’s impossible to get anything more then a third. So what’s the point of picking any strategy if whatever you do seems have the same outcome by Frank?

So you are fed up with thinking as there is no need to do anything other then throw paper over and over. But all of a sudden, Frank starts to play scissors! From this switch, he suddenly moves to a 100% win rate and causes you to lose all your money. This is called exploiting. Frank deviated from his optimal strategy in order to exploit and take advantage of your suboptimal plays. Knowing this you decide you want to make him taste a bit of his own medicine by exploiting his suboptimal tactic you decide to try rock. Your smirk turns into surprise as you realize he chose paper this time. Go home he says, you’re too dumb.

Donkey Spaces

Donkeyspace² refers to the space in which a suboptimal tactic is used to exploit another suboptimal tactic. An even slyer definition of donkeyspace refers to the space in which using a suboptimal tactics is used to encourage a suboptimal tactic to exploit the original tactic, only to use a suboptimal tactic to exploit that suboptimal tactic. Talk about inception.

Even in high level tournaments or matches, professionals constantly enter this donkeyspace and this is were the whole “mindgame” meta comes from. Before we get too far, I like to define mindgames as a more general definition of donkeyspace, which includes external interactions such as a players habits and psychological states. Donkeyspace is closer to a theoretical approach where you have thought of the suboptimal plays and knowing that in a clash of two specific plays will yield a positive pay off to you. Mindgames do not necessarily need to have a mechanical goal in which you end up with the edge, but rather incite and bait the opponent until you can exploit this weakness. While both donkeyspace and mindgames require you to understand the current state of an opponent, I prefer donkeyspace as using a players knowledge against them and mindgames as using their own psychology against them.

It is rare to see optimal players make it to the top in any tournament, and this is because these players are so entrenched in the game mechanics and theory they don’t see the human interaction and fail to take advantage of plays that would yield in victory. If you know a person always tends to paper, why are you falling on an optimal strategy when the “best” strategy is actually to play scissors? Is it because they know you know they prefer paper and will pick rock just for that one special time?³ Optimal players avoid risk and this is one of the points that sets them back from winning tournaments. They aren’t planning to win, they are concerned with not losing.

Extension to Vanguard

Theory and practice always have conflicting points. When playing against people and using donkeyspace keep this in mind:

Theory:

1. Always assume the opponent plays optimally.
2. Always assume your opponent is an expert in navigating donkeyspace.

Practice:

1. Your opponents are all idiots.
2. They are too dumb to be mindgamed.

And sadly, this is quite true in vanguard. Don’t try to do fancy stuff to lead the opponent into a maze of decision trees. More often then not it’ll go right over their heads and they just to the same thing they have always done. They lack both the knowledge and the adaptability to take what you give them. The key is to figure out your opponent skill level and judge whether or now he will react when presented with a suboptimal play. And vice versa don’t get too hung up when your opponent consistently makes poor choices, just take advantage of them in a straightforward manner until they learn or lose without worrying about potential “traps”.

I find that playing on a donkeyspace or mindgame level a really satisfying thing, as the constant baiting and provoking as well as out-thinking the opponent really plays into account here. You have all the tools at your disposal and you really are playing the game to the best it can be. You can predict the opponent and counter their counter to your plan. You aren’t blind to your choices and what results from it and you do feel that you have control over the game. But this can’t be reached unless your opponent also has the knowledge and experience to match up. While it’ll be great to have all players to play like in “theory”, the tournaments and attitudes voiced by the majority currently falls right into the “practice” ideology.

Absolutes or Nash Equilibrium

Much of the later part of this article focuses on using and abusing suboptimal plays in vanguard, but I need to point out a couple of things that can’t be played suboptimally because you gain no situational advantage for doing so. If I

All decks should have 12 Crits and 4 perfect guards⁴. There is not one set of triggers that play better against another set of triggers then 12 crit. It is strictly:

• Tier 1: 12C 4H
• Untiered: everything else

Same case for perfect guards. There are still no builds viable enough so far as to even consider quintets.

I guess other ones would be to ride to grade 3 as fast as possible and put crits on vanguard if they are at 4 damage and you can pass, and other small things.

Exploiting terrible players

The format will be divided into the particular suboptimal tactic your opponent has, what is “optimal”, what “suboptimal” play can be subjectively considered superior, and details as to why. I invite you to think of the optimal and suboptimal decisions before looking through it.

Scenario

Your opponent is not running the optimal lineup (Something like 8C/4D/4H). You are at 4 damage and have enough to guard all his attacks. You have a good chance of winning if you tank for a couple of turns, but if you decide to let his vanguard through you’ll have a better chance of winning with the cards you saved. Your opponent initiates an assault on your vanguard with no additional crits.
Your plan is to…

Optimally: Guard it

Sub-Optimally: Not guard it

I guess one can start by arguing I never specify how much better not guarding the attack actually is in the question. But we can look at winning plays based off of two distinct directions. The first is having the correct pieces for a extensive finisher such as Bad End+Ogre or Immortal+Samurai. But that shows a complete polarity, a 30# chance of losing and 70% chance of winning (since no deck can survive a full zerg rush). Had you wasted cards to block the opponent’s attack not only did you drop a 70% win rate but your proceeding rush may not be as soundly unstoppable as it was before. The second way are viable finishers, akin to restanding vanguards and giant pumps. They aren’t the all or nothing plays as in the first example, but they uniformly applicable to present and future. The success of these finishers is to attack at a point where the opponent does not have enough defense, and as we need to predict a 70% they pull non-crits (read, 5k bodies) that leaves an opponent where it could lead to your favour. Hence, based on the information you should know I’m dealing with the second case.

Your opponent has an astounding (1 - 41/49*40/48) 30% to get at least one crit. I mean, that’s almost one in every three games. Surely you wouldn’t risk it by letting it pass. Your hand looks decent enough to survive a couple of turns and you aren’t guaranteed to win next turn either.

But this is where randomness plays out. Neither you nor your opponent is going to know exactly what is going to happen in next three turns. Optimal takes into account the average of those three turns but not the precise number. So what if you start to pull below average and your opponent gets above average? It is absolutely in the realm of probability that you can lose.

But there is also something inherent in the way the trigger ratios are presented. The 4 draw trigger allows them to succeed in very long battles. The reason why 12 Crit is superior is due to the game favouring offense more than defense, and the mean number of turns it’s advantage starts to decay is sometime after natural game speed. But if you keep holding back with 12 crit then you’ll start to lose a the battle of attrition. Remember you have a 43% chance to break out the crits so the advantage is clear.

You might be asking why guarding it is still optimal given my above point. That’s because 30% is still a fair amount of risk, provided that tournaments are best of 1 or 3 (depending on the format). It would be on average more advantageous to you to keep going for a few more turns, as an opponent that lacks access full crits troubling in other departments. But playing suboptimally lowers the amount of chaos or “randomness” remaining and closer to a set of micro-scenarios where theory takes a greater hold.

Scenario

You are stocking a full field. Your opponent has all but a side booster, but uses his sole stage 1 rearguard to attack your grade 2 rearguard. You don’t have a replacement attack on hand but you still have a booster to guard it. Your plan is too…

Optimally: Guard it

Sub-Optimally: Not guard it

Let’s start with the net advantage of the two possible scenarios. If you get an attacker the turn after then you lost nothing (if you consider that the RG attacked the VG, then you would need \5/ or an interceptor amount of shield). But if you fail to pick up an attacker you lost an important 2-stage attack. Even if you have no \5/ to stop the rearguard attack, a \10/ would only cause you to lose 5k rather then a full 10k. When we add in the probabilities then the solution is clear; Assuming a grade ratio of 8:11:14:17 you have a 38% of the time to draw an attacker. If you happen to have a set of grade 1 units that can be substituted for then it could be 47%. Still, when compared to the higher 62% percentage of getting that -5. Summarized:

Not Guarding: (0)(19/49) + (-10)(30/49) = -6.122
Guarding (min): (-5)(19/49) + (-5)(30/49) = -5
Guarding (max): (-10)(19/49) + (-10)(30/49) = -10

The reason why you would consider a decision such as this is dependant on the deck. Or specifically what your ‘replacement’ could be. Unfortunately most of the good rearguard effects are only for grade 2’s so your opponent can take out two birds with one attack. So this only works when your opponent is attacking a weak or empty grade 2 so you can pull a legion mate or some other card to replace it. In contrast a legion mate can be sacrificed for re-legioning. If the legion mate has a raw advantage over a temporary attack advantage then swapping it for a beatstick can provide a solid rush.

So far I’ve been hammering you with what irrational plays you can make in order to secure some other sort of advantage. Yet I haven’t asked for any situation where you would force an opponent would make an irrational play. Now we flip the scenario around and propose the question of what you will do when you could send a 1-stage at the rearguard. You want your opponent to not guard the attack. To do so, you should target the grade 2 rearguard with the lower threat. It might seem irrational as well but you are opting to cause the opponent to be irrational as well. It’s a interesting gambit which requires you to understand an opponent’s reactions corresponding to what they have available in their hands.

Scenario

Take the previous scenario, and change one of the following: Either you have a grade 1/3 unit being attacked instead, or the opponent is throwing a 2-stage lane. Your plan is too…

Optimally: Not guard it

Sub-Optimally: Guard it

A bit of math churns out: 5 * 19/49-5 * 30/49 = -1.122

And against a full -5, it hurts to consider dropping it. The sub-optimal clause is described in the first example, where you aim to reduce entropy in order to secure a quicker game. I believe you can work out the kinks in the solution given the former examples.

New blog and plan moving forward

• Brampton Booster
• Jan 03, 2015
• Comment

We are back in business! This is the new location of my old Brampton Booster. As you may or may not tell, there are a lot of exciting details for everyone. The ones that would concern you the most are:

• Faster loading times. Wordpress and other bloatware trimmed out to speed up blog.
• No Ads. This goes hand in hand with faster loading times, but now you have no obstructions in reading solid articles
• IntenseDebate comment system. Has a ranking system and smart threading to make sure good discussions stay on top.
• Interactive elements. Check out the Hypergeometric Calculator on the side, or the program below.
• Open source. The blog is hosted on Github, so just head over to my repo to suggest changes or use ideas for your own blog.

My philosophy is having informative and expansive content in a straight and legible content being most important for readers. Informative is obvious, as that’s what blogs should be made for, and expansive because I want to impart learning as well. While to-the-point content is critical I find it equally important that readers find what they need to find quickly (the legibility part). This is why my blog has a small navbar and quick access elements so you should be only 2 clicks away from every single article I publish¹.

1. Interactive elements

In most blogs you just read text, view images and maybe watch a video or two. I want some form of involvement from the reader (if they feel up for it of course). The goal of this blog is to learn the theory and practice it. Once you understand it, you can apply it in all sorts of ways and converse with a larger group of people. Principally you should never trust me! Do your own math and prove it yourself!

So one of the ways I aim to implement proactive learning is providing tools for you. For example, let’s take the Hypergeometric Calculator on the right. I say that the chance to get at least one trigger from a twin drive check is approximately 55%. Can you verify this is in fact correct? Well you know a standard deck contains 50 cards, with 16 of them triggers. So you can put z=50 and and w=16. Twin drive involves removing 2 cards from the deck so y=2. Lastly we want at lease 1 trigger so we set u=1 and hit <pre>Evaluate</pre> and get “Chance >= 1: 54.2%”. Pretty solid. Now try it at turn 3 where you are expected to have 36-38 cards and 11-13 triggers.

Hell, you might not even use it for card games. Say 80% in your class of 30 had a cold and you want to pick out 3 students. If you put in the numbers correctly, then almost half the time all 3 students would have a cold! ² So you can play around with this and see different percentages. You can then look at the source code and understand how the answer is evaluated. Or you can search online for a different site and compare values. So have fun and learn the math at the same time.

2. Testing Database

Being open and transparent is necessary for trust. There are many people who put time and effort into meticulously testing many different decks and don’t want to have their results taken at face value. I am currently developing a program that will track every game played and its various notes, so you can expect to find quality information before you decide to invest money into a specific build.

While it will start out restricted to invitation only (in terms of adding to the database), I hope to release a public key so anyone willing can contribute to the testing. However, everyone is able to view the results of the the cardfights. Given some database experience you can send specific queries by Python integration to get detailed information on whatever it is you are looking for.

Since most of the population do not deal with databases every once in a blue moon I’ll post neat graphs that display expected and unexpected trends in the decks tested. All of this is backed by a secure server and frequent backups and more importantly, people who volunteer their time into improving the vanguard community.

3. Reader Input

And of course if anyone has any suggestions they want to make do not hesitate and do send the love (or hate) my way. It makes you feel good, it’ll make me feel good ³. I know the vanguard community is stagnating a bit maybe because you are being constantly let down by Bushiroad but the game and its design is great and you should thank whomever for crafting such a unique set of mechanics ⁴ that was not a stage for promoting all the anime they sponsor (not hating on Weiss Schwarz of course)

And as it shall, the comment box is right below.

The Great Resolution

• Brampton Booster
• Sep 16, 2014
• Comment

Clunky resolution systems are those with a lot of repetitive and redundant nature to it, and require constant attention and memorization to both players. When combined with poor effect text, symbols and structure it can make any idea fall apart. Out of all the card games I have been playing (including Online CG that’s about a dozen) I admire Cardfight!! Vanguard’s brilliantly thought out rule set, and after 3000 different cards there has been no more then a couple of line changes to accommodate new mechanics ¹. All rulings are made by reading the handbook line-by-line. In comparison; MTG is the largest card game and houses an equally large 200 page rulebook. The runner-up, Yugioh, has dug itself into a giant hole by deliberately avoiding clarification on conflicting rulings and leave to the the floor judge to make the best of it. Vanguard is by no means the best, perfect model for resolutions. And the other resolution systems are great models as well with many benefits. Nonetheless, CFVG is a brilliant model to study and develop from. Of course, you can’t take my word for it. So I’m going to go through the different kinds of systems and explain the flaws and improvements they’ve made from previous iterations of resolution design.

To be indefinitely board and inaccurate I’m going to define a card effect structure in three parts:

• Trigger (Red)
• Condition (Yellow)
• Result (Green)

If you are hoping to learn the details of actually playing these sample games, Ignore everything I write down and instead point to the references provided at the end of each section. Not that it’s wrong, but rulesharks exist to make you fall into infinitesimal loopholes and penalties. As I have the most experience with MTG, YGO and CFVG I’ll cover those primarily. You can thanks Blaise for indirectly aiding me to add one additional section.
The LIFO stack

I’ll take the 6th definition from wikitionary’s database to describe what a stack is:
(computing) A linear data structure in which the last data item stored is the first retrieved; a LIFO queue.
LIFO
(computing, accounting) Last In First Out (describing a stack data structure).

This is the most prominent standard in TCG’s. The core aspect relies on this:

1. Play Card A. A goes into the “stack”.
2. Play Card B. B goes into the “stack”.
3. Play Card C. C goes into the “stack”.

To fill a stack and this:

1. Take Card C out of the “stack”.
2. Take Card B out of the “stack”.
3. Take Card A out of the “stack”.

To empty the stack. The key thing to note is that you can not take Card A out of the stack before Card B is taken out. And Card B can’t be taken out before Card C. The Card on the bottom can’t be removed before taking out the ones on top.

MTG

FYI, spell := Card

To play a spell in MTG, you first must meet the Condition.

[+]

• If you just played a spell, it is added to the stack, and you can either play another spell or let the opponent play a spell.
• If your opponent just played a spell, it is added to the stack, and they can either play another spell or let you play a spell.
• If neither player wants to play a spell (rather, both players consecutively ‘let’ the other play), then the stack empties.

However, the stack isn’t just dumped back out immediately like pudding. It goes one by one; a spell is taken out of the stack, the Result happens, and the active player ² can once again choose to play another spell (and hence goes back to [+]). Both players have to once again decline to play a spell before it the next spell in the stack Results.

Continuous effects are like “rules of the game” once in play. You would continue playing as if the game always had that rule. If you played it during a stack, once the Result of the card has past you treat the game as if the rule always existed.

Now we get to “counters”, which is really why WoTC ³ decided upon a LIFO system. On the resolution of spells that “counter” another spell, the spell chosen to be targeted has all of its text removed, as if it is a completely blank card. When the stack reaches to that card, it ditches the card. Counters exist to stomp and roll all over an opponents plan. Say there was a card who’s Result would win the game. You don’t want that to happen so you play a counter spell to prevent that card from Resulting, therefore buying you time (possibly enough to win the game yourself). This miracle/comeback play is necessary to keep games dynamic and unexpected. If you think about it, it is possible to categorize effects into three areas: those that start a stack (the seed), those that are played in response to a stack (the counter), and those that do not start a stack or can be responded to (the passive/continuous).

For the finer details of stack and priority thank Yare for this well written article:

As the activate player, you can cast a spell every step of the stack resolution. Asking for each iteration being forced to announce every decision is incredibly tedious. A stack could be near the end and you can decide to cast something and build another long stack, making the constant unpacking and packing of cards is difficult to track. Long stacks are uncommon as it requires a large amount of mana and cards. But if you cast a spell that let’s you draw cards / add mana, then you grow the chain (because you get a chance to play every time a spell resolves). Have enough of this and you can get infinitely long chains. The MTG engine does not promote huge hands, extensive drawing capabilities or infinite mana, to combat the possibility of these large/long stacks. It does however, limit the growth of resources and therefore makes the game exceedingly long. A lot of this game is spent waiting to gain enough mana, cards, waiting to toolbox, draw non-land.

So the big problems MTG resolution system has are:

• Large memory strain for ordered stack events
• Redundancy in saying no, especially for long stacks
• Constant peckering of both players
• Resources are forced limited to reduce stack length ⁴

YGO

FYI, chain:=stack

To activate a card in ygo, you first must meet the Condition.

• If you just activated a card, it is added to the chain, and you can either activate another card or let the opponent activate a card.
• If your opponent just activated a card, it is added to the chain, and they can either activate another card or let you activate a card.
• If neither player wants to activate a card (rather, both players consecutively ‘let’ the other activate), then the chain empties.

I’d like to point out that Yugioh cards have a “Spell Speed” which acts like a miniature condition. You are not allowed to add to a chain if the Spell Speed of the Card you want to activate is lower than the Spell Speed at the top of the chain.

Just like before, the chain resolves one by one. Unlike stacks, chains do fall like pudding and all the Results happen one after the other with no interruptions. Here is where we get interesting. The Result or Condition of a Card could Trigger a different Card. It could Trigger multiple Cards. So when do we get a chance to apply its Condition or Result?

The simple answer is when the Result of the last Card in the chain is done. But then how do we determine the order of all these Cards? YGO has implemented SEGOC ⁵ rules to resolve this issue. The order basically takes all the Cards that have Triggered and allocate them on a new chain based on a few rules.

Continuous effects also fall into the SEGOC rulings, so the Result or Rule the card would implement will not start until all the chain has finished (but before SEGOC clause happens). Note that this isn’t a real solid rule, as there are two rulings about these effects that conflict each other, but effects in this way make it more consistent to the practice established.

For more information about SEGOC try this article about the resolution of two similar effects

Or, you may explore the entire blog

Unlike MTG, YGO tried a new method by forcing chains to completely resolve before play resumes. That cuts out “I choose not to cast” by over 70% ⁶. This also stops chains from being refilled constantly making each chain much shorter on average then stacks.

But SEGOC and Triggers start the same deadly loop. At the end of a chain, a new chain starts with all the Triggered cards put into a new chain, which CAN be included in. So YGO just splits one massive chain into a series of smaller ones. Unfortunately, since the entire chain resolves, having copious amounts of cards that draw or add more cards into play will refresh stocks every time. In MTG by the time you get down to a particular spell the available the conditions and spells in the stack have changed. In YGO it starts all over so while you have a different scenario the abilities in play are all there. So you can tell YGO has to have the same restrictions as MTG to demote extensive chaining. I should also say that users barely have an option of how to order the different effects into the new chain.

So what problems did YGO solve or create?

• (+)Memory strain is much less
• (+)Redundancy is improving
• (+)Less peckering
• (-)Multiple chains that have no optional order
• (-)Limited Resources to reduce continuous chains
• (-)SEGOC Chains have limited user input

Although this last point does not concern the point I’m getting at, YGO has done a significant improvement in the understanding of card effects, now making them both consistent and logic-based.

FCBF

So YGO and MTG each stack and chain off of each other ad infinitum, which forces their cards to cut significant amounts of resources. Buddyfight aims to shorten the unnecessary drive with a 1 counter clause. Therefore, using cards now works like this:

 The turn player chooses to use one card or ability
 The non-turn player may choose to use one Counter
 If they do
 	The turn player may choose to use one Counter
 	If they do
 		Resolve the turn player's Counter
 		Resolve the non-turn player's Counter
 		Resolve the turn player's card or ability
 	If they don't
 		Resolve the non-turn player's Counter
 		Resolve the turn player's card or ability
 If they don't
 	Resolve the turn player's card or ability

Likewise, a MTG tower would be like:

 The turn player may choose to play a spell
 	If they do
 		The turn player chooses to play a spell
 		If they do
 			The turn player chooses to play a spell
 			If they do			
 				etc..
 		If they don't
 			The non-turn player may choose to play a spell
 			If they do
 				The non-turn player may choose to play a spell
 				If they do
 				etc,
 	If they don't
 		The non-turn player may choose to play a spell
 		If they do
 			The non-turn player may choose to play a spell
 			etc.
 		etc.
 	etc.
 etc.

It’s a simple and elegant solution. It doesn’t invite impressive plays, but it solves the problem of large complicated loops.

As it is so simple, my debriefing ends in exactly two sentences due to having nothing to talk about.

So what problems did FCBF solve or create?

• (+)Almost no memory strain
• (+)No redundancy
• (+)Almost no peckering
• (-)Stack has very little input
• (-)Combos become impossible due to simplicity

The cache

So we have folly’d with this “ordered” resolution for a long time. After all, it works and everyone is content. We want combos and counter-play, and sacrificing that causes people to feel like the game is overtly simple and childish. That isn’t absolutely true, but it certainly reduces the depth of the effects that effects have.

So this is where I show what neat tricks Vanguard pulls to get the best of both worlds. We start with the definition of a cache:

(computing) A fast temporary storage where recently or frequently used information is stored to avoid having to reload it from a slower storage medium.

Notice that in a cache, order is unimportant.

CFVG

To activate an effect, it has to meet it’s conditions.

1. Once you activate it, you Resolve it.
2. Then for all effects that meet the Trigger, you add those effects into the cache.
3. The activate player choose one effect that belongs to them and Resolves it.
4. Then for all effects that meet the Trigger, you add those effects into the cache.

3 and 4 continues until you have no more effects left that is yours. It then becomes your opponent’s turn to resolve all of his effects, until he is done. It keeps going back and forth until the cache is empty.

Counters do not exist in the game, and continuous effects come into play as soon as they are put on the table (they do not go into the cache nor Trigger any effects)

WOW. That is really different from any other card game out there. Let’s see what problems it solves:

• It reduces 100% of the “not choosing” plaguing card games. Redundancy is completely obliterated.
• It reduces the need to constantly flip between two players
• Card Condition and Result activate immediately. Exactly what you would naturally expect ⁷
• And unlike FCBF’s solution, it does not simplify the game or reduce combo potential.

The biggest or most obvious part about all this is the removal of counters. But actually, you can still create counter effects (When your unit is retired, call it to {R}) that are actually more interesting than normal counters. MTG/YGO counters are more like a stand off doing almost jack squat, or firing bullets at a Gundam shield. Vanguard is dynamic by letting you succeed, only to be caught by a surprise resurrection or counter attack. You can pull off your dream combo instead of it being violently stuffed by a single spell, and such counters do not merely neglect an action but turn the disadvantage into an advantage in another way (When your unit is retired, send a card from your deck to {Drop})

So what problems did CFVG solve or create?

• (-) Some memory strain
• (+) No redundancy
• (+) No peckering
• (+) Combos are completely free and malleable

Based on this small list it seems Vanguard has solved absolutely everything and there is little need to iterate further. However, there is a really really big hole in this.

Let’s have 2 card with these effects;

AUTO(R): During your End Phase, When you draw a card, you may pay the cost. If you do, this unit gets +1000.

AUTO(R): During your opponent End Phase, When one of their units gain power during the End Phase, your opponent draws one card.

Based on the way vanguard works, this can result in an infinite loop, provided there are enough cards in the deck to support the pair. While I documented a 2 card case, it could be viable for any number of units acting together. So that forces most cards to have a counterblast cost or some other cost to make sure if never repeats itself. (If have to say, that is a dumb effect above but it was only used to get the point across).

So if you want to get picky, here are some problems it suffers.

• No counters to actually stop plays, therefore creating unstoppable wins ⁸
• All effects should have to prevent self-occurring loops
• Logic base language does not work with deformed grammar ⁹

Conclusion

So we had a short article here discussing the various ways to resolve multiple effects, optimally keeping it consistent, open to flexibility, reduces redundancy, and reduces load on memory for more important tasks. While there is no prefect system as so far, nor have I talked about any horrendous ones, the ones I have mentioned are all successful games. So while a well-defined rule set does not correlate to popularity, we know that people are willing to accept imperfections and devise short-cuts in order to enjoy the game keeping the rule sharks out.